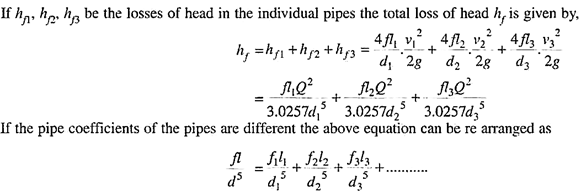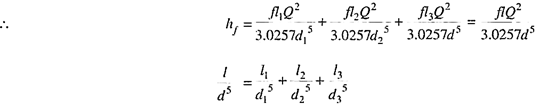Flow through Pipes in Series and Parallel: Difference Diameters, Equations and Solved Problems

Flow through Pipes in Series and Parallel: Difference Diameters, Equations and Solved Problems!

Pipe in Series:

Pipes are said to be in series if they are connected end to end (in continuation with each other) so that the fluid flows in a continuous line without any branching. The volume rate of flow through the pipes in series is the same throughout.

Suppose a pipe line consists of a number of pipes of different sizes and lengths. See Fig. 13.37.

Let d₁, d₂, d₃ be the diameters of the component pipes.

Let l₁, l₂, l₃ be the lengths of these component pipes.

Let v₁, v₂, v₃ be the velocities in these pipes.

Pipes connected in continuation as in this case are said to be connected in series. In this arrangement the rate of discharge Q is the same in all the pipes. Ignoring secondary losses the total loss of head is equal to the sum of the friction losses in the individual pipes.

Equivalent Pipe Corresponding to a Given Set of Pipes in Series:

Let d₁, d₂, d₃ be the diameters, and l₁, l₂, l₃ be the lengths of the various pipes in a series connection. Let Q be the discharge. Let h_f be the total loss of head.

Let d be the diameter of an equivalent pipe of length l to replace the compound pipe to pass the same discharge at the same loss of head.

The above relation is called Dupuit’s equation.

Equivalent Length of a Pipe with Intermediate Fittings:

Suppose a pipe of length I is provided with an intermediate fitting due to which the loss of head = k(v²/2g). Let I_e be the length of pipe, the friction loss due to which is equal to k(v²/2g).

Pipes Connected in Parallel:

Pipes are said to be in parallel when they are so connected that the flow from a pipe branches or divides into two or more separate pipes and then reunite into a single pipe.

Suppose a main pipe branched at section 1-1 into two pipes of lengths l₁ and l₂ and diameters d₁ and d₂ and unite again at a section 2-2 to form a single pipe.

Then the two branch pipes are said to be connected in parallel.

In this arrangement the total discharge Q divides into components Q₁ and Q₂ along the branch pipes such that –

Q = Q₁ + Q₂

In this arrangement the loss of head from section 1-1 to section 2-2 is equal to the loss of head in any one of the branch pipes.

h_f = h_f1 = h_f2

Hence the total discharge Q divides into components Q₁ and Q₂ satisfying the above equation.

Similarly when a number of pipes be connected in parallel, then also, the total loss of head in the system is equal to the loss of head in any one of the pipes.

For example in the arrangement shown in Fig. 13.39, the total loss of head in the system