Consider heat conduction through a hollow sphere of inner radius r1, outer radius r2 and made of a material of constant thermal conductivity. The inner and outer surfaces are maintained at constant but different temperatures t1 and t2 respectively. Geometrical symmetry indicates that the heat flow is limited to radial direction only. Further if temperature t1 at the inner surface is greater than temperature t2 at the outer surface, the heat flows radially outwards.
Consider an infinitesimal thin spherical element at radius r. Let thickness of this elementary ring be dr and the change of temperature across it be dt. Then invoking Fourier law of heat conduction.
Separating the variables and integrating within the prescribed boundary conditions
The expression for steady state temperature distribution in a spherical wall can be set up by integrating the Fourier rate equation between the limits –
(i) r = r1 where the temperature is stated to be t1
(ii) r = r where the temperature is to be worked out
Quite often it is considered advantageous to write the heat flow equation through a sphere in the same form as that for heat flow through a plane wall. Then thickness δ will be equal to (r2 – r1) and the areas A will be an equivalent area Am. Thus-
Comparing equations 3.22 and 3.23,
Am = 4πr1r2 … (3.25)
Further, Am = 4πr2m = 4πr1r2
ADVERTISEMENTS:
Obviously geometric mean radius of the spherical shell is,
rm = √r1r2…(3.26)
Spherical composites are dealt with in the same way as composite walls and cylinders.
The heat flow through a three-layer composite spherical shall will be:
Further, if the convective film coefficients at the inner and outer surfaces of the composite spherical shell are also considered, then-
Example 1:
The following data pertains to a hollow cylinder and a hollow sphere made of the same material and having the same temperature drop over the wall thickness.
ADVERTISEMENTS:
Inside radius = 0.1 m and outside surface area = 1 m2
If the outside radius for both the geometries is same, calculate the ratio of heat flow in the cylinder to that in the sphere.
Solution:
Let suffix 1 and 2 refer to inside and outside surface respectively.
Example 2:
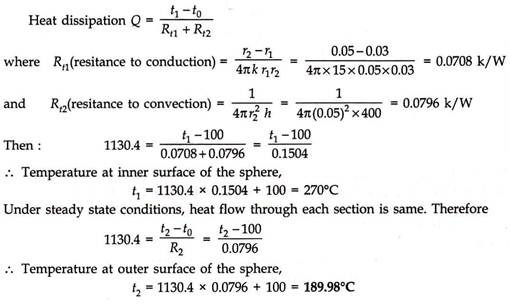
A hollow sphere of inner radius 30 mm and outer radius 50 mm is electrically heated at the inner surface at a rate of 105W/m2. At the outer surface, it dissipates heat by convection into a fluid at 100°C and a heat transfer coefficient of 400 W/m2 K. Determine the temperature at the inside and outside surfaces of the sphere.
It may be presumed that there is no energy generation and the thermal conductivity of the material is constant at 15 W/mK.
Solution:
r1 = 0.03 m and r2 = 0.05 m
Rate of heat dissipation Q = q × 4π r12 = 105 × (4π × 0.032) = 1130.4 W