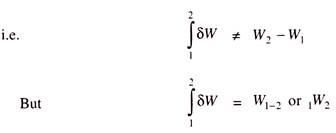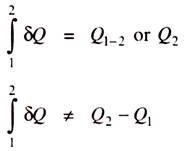Thermodynamic Work: Equations, PdV-Work, Heat, Pressure and Temperature Measurement. In this article we will discuss about how to measure work, heat, pressure and temperature. Learn about:- 1. Mechanical and Thermodynamic Work 2. Equations for Work Done in Various Processes 3. PdV-Work 4. Heat Measurement 5. Pressure Measurement 6. Thermometers and Measurement of Temperature.
Contents:
- Mechanical and Thermodynamic Work
- Equations for Work Done in Various Processes
- PdV-Work
- Heat Measurement
- Pressure Measurement
- Thermometers and Measurement of Temperature
1. Mechanical and Thermodynamic Work:
ADVERTISEMENTS:
W.D. = F x dl
When a force F acts on a body and causes a displacement through a distance in the direction of force, then the work is said to be done and this work is equal to the product of force and distance moved.
i.e., Work done = F x dl
If F is in N, and dl is in m then the resultant unit will be Nm or Joule.
ADVERTISEMENTS:
“It is an interaction between the system and the surroundings and the work is said to be done by the system on surroundings, if the complete external effect is lifting up of the body”.
Let us consider a battery and motor system . The motor is driving a fan. The system is doing work on the surroundings.
When the fan is replaced by a pulley and weight, then the weight may be raised as the pulley is driven by the motor. Then the complete external effect is raising up the body, so the system is doing thermodynamic work.
ADVERTISEMENTS:
When the work is done by the system, then it is taken as + ve, and when the work is done on the system, it is taken as – ve.
Work is a Path Function and Properties are Point Functions:
It is possible to take a system from state (1) to state (2) by following many quasi-static paths such as A, B, C etc:
Now if we consider the path 1-A-2, then the work done during the process 1-A-2 will be equal to the area under the curve 1-A-2 and if we consider path 1-B-2, then the work done during the path 1-B-2 will be equal to the area under the curve 1-B-2.
ADVERTISEMENTS:
So, the work done during the process directly depends upon the path and not on the end states. So, work is called Path function and δW is an inexact or imperfect differential.
Thermodynamic properties are point functions, since for a given state, there is a definite value for each property. During change of states, the values of the properties will change and this change in values of the properties does not depend on path, but it depends upon end states and hence all the properties are point functions.
The differentials of point functions are exact or perfect differentials and the integration is simply, e.g.,
Operator δ is used to denote inexact differentials, operator d is used to denote exact differentials.
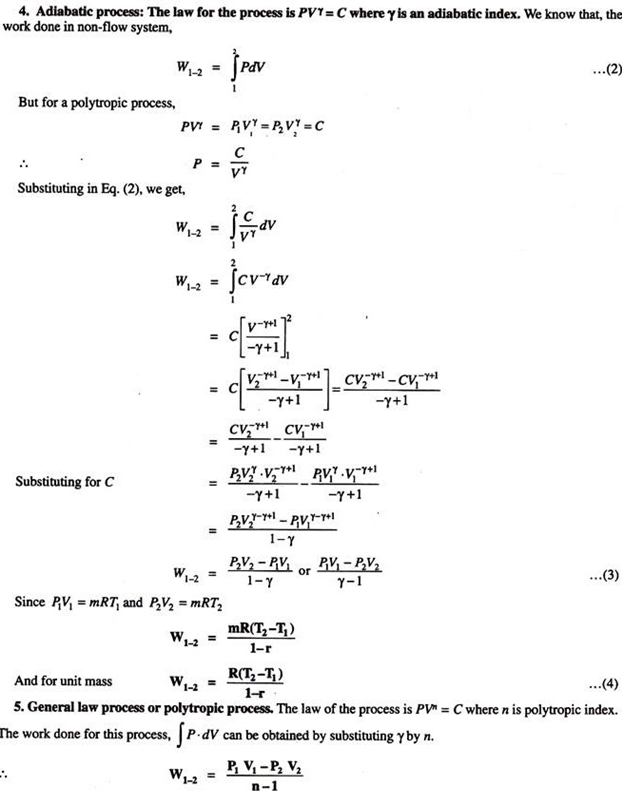
2. Equations for Work Done in Various Processes
:
PdV Work in Various Quasi-Static Processes:
3. PdV-Work:
Consider a system consisting of a gas in a cylinder fitted with a piston. During the initial condition of the piston i.e., when the piston is at position (1), the pressure inside the cylinder is P1 and volume is V1. Let the gas expands as the piston moves to position (2). Then the pressure falls to P2 and volume will increase to V2.
Now, consider a small movement of the piston dl during which pressure P is assumed to be constant. If a is the cross sectional area in m2, then the force acting on the piston is given by –
F = P x a
And the small amount of work done by the gas on the piston is causing the displacement
The initial and final states of the system can be represented on PV- diagram. The work done W1-2 will be equal to the area under the curve 1-2 on the PV-diagram.
Note:
Here the piston is assumed to move very slowly so that path 1-2 is Quasi-static (i.e., nearly static) and every state passed through is an equilibrium state.
4. Heat Measurement:
“Heat is a form of energy, which crosses the system boundary due to the temperature difference between the system and the surroundings”.
When the two bodies, one hot and the other cold are kept in contact with each other, then the hot body loses heat and becomes colder and the cold body gains heat and becomes hotter and this process continues till the thermal equilibrium is reached. At this stage the two bodies will be at the same temperature.
Like work, heat is a path function and we know that the differentials of path functions are imperfect differentials. If Q is the heat transfer, then the magnitude of heat transfer during the process 1-2 is given by,
Note:
When heat flows into the system then it is taken as +ve and when heat flows out of the system then it is taken as –ve.
5. Pressure Measurement
:
It is defined as force per unit area i.e.,
P = F/A
F = Force in newton (N)
A = Area is a m2
Then the unit of pressure will be N/m2, which is the basic unit of pressure, in SI units. This is also sometimes called as Pascal (Pa). Since this unit is very small, when compared to many engineering values, the units like, KPa, MPa, bar are used.
1 bar = 105 N/m2 = 100 kN/m2 = 100 kPa
Pressures are also measured in mm, or cm, of Hg or H2O column. The pressure exerted by the atmosphere is known as atmospheric pressure and is denoted by 1 atm. In various units, the value of 1 atm pressure are given by,
1 atm = 760 mm of Hg = 101325 N/m2 = 1.01325 bar.
= 10.33 m of H20 = 1.033 kg/cm2
Another useful unit of pressure is 1 Torr and this is equal to pressure exerted due to 1 mm of Hg = 133.34 N/m2.
Pressure is measured by using-
1. Barometer – This measures the atmospheric pressure.
2. Bourdon Pressure Gauge – This is used to measure pressure inside the pipes/containers etc.
3. Manometers – These are used to measure pressure inside the pipes/vessels etc.
The atmospheric pressure acts both on the container and the gauge which measures the pressure inside the container.
When the pressure of the system is more (i.e., +ve pressure) than the atmosphere, then gauge pressure is +ve and Pabs or Preal is given by,
Pabs = Patm + Pgauge
If the pressure of the system is less (i.e., s -ve pressure) than the atmospheric pressure, its gauge pressure is –ve and it is known as vacuum and,
Pressure Exerted due to a Column of Fluid:
Now consider an elemental vertical cylinder of cross sectional area a.
Then we know that-
6. Thermometers and Measurement of Temperature:
Thermometric Property and Thermometers:
Temperature:
It is property of the system or of a body which distinguishes a hot body from cold one.
Thermometers:
In order to obtain, a quantitative measure of temperature, a reference body is used, and a certain physical characteristic of this body which changes with the temperature is selected. The selected characteristic is called the thermometric property and the reference body which is used in the determination of temperature is called thermometer.
A very common thermometer, consists of a small amount of Hg in an evacuated capillary tube. In this case the change in length of Hg is taken as the measure of temperature.
There are five different kinds of thermometers, each of them have their own thermometric properties viz.,-
Principle of Temperature Measurement:
Let x be the value of any thermometric property as given in the table and θ be the temperature corresponding to x.
When the temperature changes, the values of the thermometric properties change proportionately. Therefore the value of temperature is proportional to the values of thermometric properties.
Thus for any change in value of x (i.e., Thermometric property) the temperature θ can be found out. Thus from this equation, we can construct a scale and calibrate it to give us direct temperature readings. Here it is to be noted that xt is the value of the property at triple point and is constant.
A scale of temperature is based on some reference or fixed point to which an arbitrary value of temperature is assigned. Thus on a thermometer with some fixed or reference point, any temperature has a unique numerical value w.r.t. the fixed reference point.
(1) (a) Celsius scale, (b) Fahrenheit scale.
(2) Absolute Temperature scale.
(3) Ideal Gas temperature scale.
1. (a) Celsius Scale:
This scale is named after Anders the Celsius, who devised this scale. There are two fixed points on this scale. One is ice point and the other is the steam point. These two points are numbered as 0 and 100 on Celsius scale. The interval between them is divided into 100 equal parts and each division, is known as one degree Celsius and degree Celsius denoted as °C.
(b) Fahrenheit Scale:
On this scale the ice point is numbered as 32 and steam point is numbered as 212. The interval between them is divided into 180 equal parts and each division, is known as 1 °F.
The temperature readings on one scale can be converted into a reading on the other scale by the following formula:
2. Absolute Temperature Scale or Kelvin Temperature Scale or Thermodynamic Temperature Scale:
This is an internationally accepted scale. In this scale the temperature is measured from absolute zero temperature.
Absolute zero is the temperature at which all the vibratory, translatory and rotational motion of the molecules of a gas, is supposed to cease. A gas on cooling contracts in volume. In case of perfect gases, ‘Charles’ found that decreases in volume per degree centigrade decrease in temperature is 1/273.16th of its volume at 0°C and pressure remaining constant. Thus the volume of gas will be zero at temperature -273.16°C. This temperature 273.16°C below or behind 0°C (or -273.16°C) is called the absolute zero temperature.
In this scale there is one more reference point taken, the Triple point of water. This is a temperature at which all the three phases of water exists in equilibrium, and the value of triple point temperature is 0.01°C or 273.16 K at a pressure of 0.006 bar.
Here it is to be noted that, the ice point temperature on this scale is 273.15 K, and steam point temperature is 373.15 K and therefore to convert the temperature given in °C to K, just add 273.15.
K = °C + 273.15 or in general, K = °C + 273. Here it is to be noted that the value of Absolute zero on this scale is -273.16°C.
3. Ideal Gas-Temperature Scale:
This is similar to absolute temperature scale. This scale is constructed by using constant volume gas thermometer.
In a constant volume gas thermometer, gas will be used as the working substance and as all the gases behave as ideal gases at low pressure, thus scale is known as Ideal gas temperature scale. The value of absolute zero on this scale is same as that given by Absolute temperature scale i.e., – 273.16°C.